..................         

OVERVIEW
Chapter 16 - General Plane Motion of Rigid Bodies

We have already studied the kinetics of particles.
Now we will study the kinetics of rigid bodies, that is, the relations between the forces acting on the body and the motion produced. 

Our approach will be to consider a rigid body as a special case of a system of particles.  A rigid body is a large numbers of particles, rigidly connected.  We can use the results we have already obtained for the motion of systems of particles.

Specifically, we will use these 2 equations that we have already derived for a system of particles: 

ΣF = maG

where

  • ΣF is the resultant of the external forces acting on the system
  • m is the total mass of the system of particles
  • aG is the acceleration of the mass center, G, of the system of particles
ΣMG = dHG/dt
where
  • ΣMG is the moment resultant of the external forces about the mass center of the system
  • HG is the angular momenta of all of the particles of the system about G
These equations are very general:  they apply to any system of particles in a Newtonian frame. 

We will now apply them to a rigid body in plane motion.
With a bit of work we can derive the following equation: 

dHG/dt = IGα

In english this equation says: 

"The rate of change of the angular momentum about the mass center of a rigid body in plane motion is equal to the product of the centroidal mass moment of inertia and the angular acceleration α of the body"

                                                 

So we have for a rigid body in plane motion we have these 2 equations:

ΣF = maG

        ΣMG = IGα

where

  •  m is the mass of the rigid body
  • aG is the acceleration of the mass center
    (aG is the same as a with the bar on top. Recall that quantities that refer to the mass center often have a bar on top.)
  • IG is the moment of inertia of the rigid body about its mass center
  • α is the angular acceleration of the rigid body
    (IG is the same as I with the bar on top.  Recall that quantities that refer to the mass center often have a bar on top.)                                     
Note: These 2 equations have the following restrictions (that came out in the derivation which we skipped):
  1. They are restricted to the plane motion of rigid bodies, that is, to a motion in which each particle of the body remains at a constant distance from a fixed reference plane.
  2. The rigid bodies considered will consist only of plane slabs and of bodies which are symmetrical with respect to the reference plane.

 

Approach for solving problems involving the plane motion of rigid bodies:
The approach to solving problems involving rigid bodies is essentially the same as the approach we used for particles.  

  1. Draw the FBD of the rigid body. (figure a below)
  2. Set this FBD equal to the inertia vector diagram. (figure b below)
    In the case of rigid bodies this IVD will show a single effective force, maG, and a single effective couple, IGα .  
    (Recall that for particles we did not have any effective moments, just the effective forces.)
  3. Apply the two (vector) equations above in the three component directions.
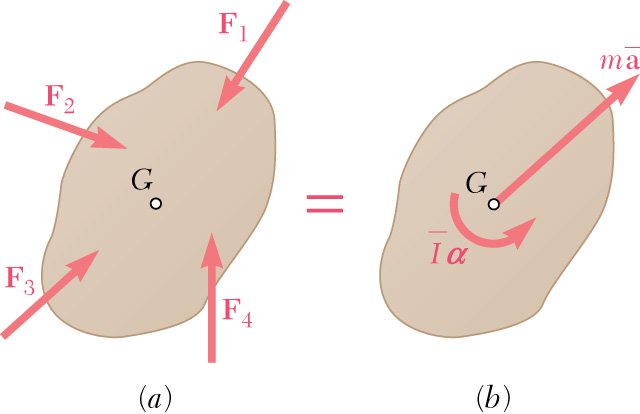
figure (a) shows a rigid body with all of the external forces acting on it and this is equated to figure (b) which shows the rigid body with the effective forces (=mass times the acceleration vector of the mass center) acting at the mass center and the effective moment (= moment of inertia of the mass center times alpha)

For a 2 dimensional problem we would have:

ΣFx = maGx

ΣFy = maGy

ΣMGz = IGα

So we can think of the motion of a rigid body being broken into two parts: a pure translation with the mass center, maG, and a pure rotation, IGα.